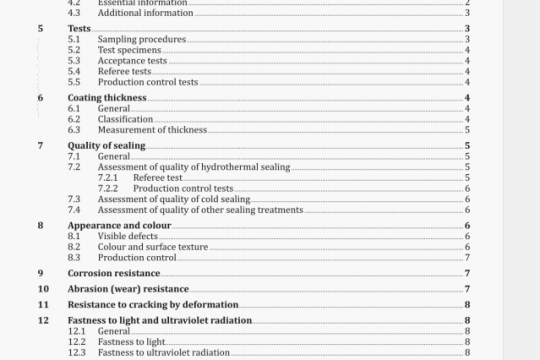
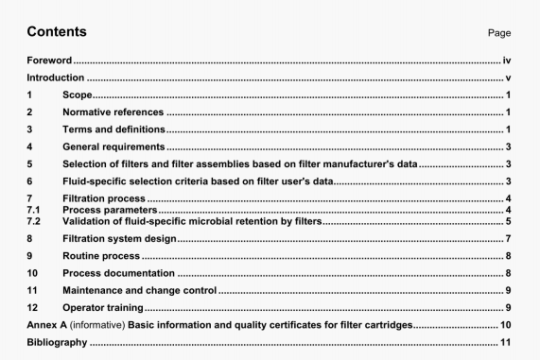
ISO 06143:2001 pdf download
ISO 06143:2001 pdf download.Gas analysis – Comparison methods for determining and checking the composition of calibration gas mixtures.
– parameters of the analytical method,
— conditions of measurement, if relevant,
— number and sequence of calibration measurements (see 5.1, steps D, E, F).
e) Perform the calibration experiment, i.e. measure the response, v, for samples of the chosen reference gas mixtures, and estimate the uncertainty u(v) of these response values (see 5.1, step G).
f) Calculate the analysis function, x = G(y), from the calibration data, using regression analysis (see 5.1, step H).
g) Examine whether the calculated analysis function is consistent with the calibration data within the relevant uncertainties. If the result is acceptable, proceed to h). If not, revise the calibration design (see 5.2.1).
h) Determine the uncertainty level of the prospective results based on the analysis function for the relevant ranges of responses and analyte contents. If the result is acceptable, proceed to i). If not, revise the calibration design (see 5.2.2).
i) Prior to analysing a prospective calibration gas sample, test for instrument drift to ensure that the analysis function is still valid for the specified analytical task (see 5.2.3). If the result is acceptable, proceed to j). If not, recalibrate the measuring system.
If the prospective calibration gas contains other components than the reference gas mixtures used for calibration, validate the applicability of the analysis function using at least one additional reference gas mixture of appropriate composition (see 5.2.4).
NOTE It is not necessary to test for drift in conjunction with every analysis of a calibration gas sample. The frequency should be based on experience concerning the stability of the measuring system.
Similarly, the composition of additional reference gas mixtures used for validation should be based on experience concerning the cross-sensitivities of the measuring system.
j) Determine the composition of the prospective calibration gas as follows:
— measure the response v,
determine the uncertainty u(v) of the response y, calculate the mole fraction x = G(v) using the analysis function determined in f), calculate the uncertainty ii(x) of the mole fraction x using the results obtained in h) (see 5.3).
k) State the result of the entire analysis (see clause 7).
In addition to determining the composition of a (prospective) calibration gas mixture, the general procedure may be used to check a pre-established composition. To this end, the mixture under consideration is analysed using the procedure outlined above, and the composition obtained is compared with the pre-established composition. Clause 6 specifies a procedure where, for each analyte concerned, the difference between the content obtained by the confirmation analysis and the pre-established content is examined against the uncertainty on this difference for significant departure from zero.
The general procedure may also be used to examine the mutual consistency of pre-established composition data for a series of calibration gas mixtures or reference gas mixtures. Clause 6 specifies a procedure where, for each analyte concerned, the measured responses and the pre-established analyte contents of all calibration gases under consideration are tested for compatibility with the known response behaviour of the measuring system.
NOTE 2 The individual weighted differences can be used as a diagnostic tool for identifying potential outliers among the calibration data.
In addition to the procedures described above, every calculated response curve has to be inspected visually. This visual inspection is necessary to reveal “nonsense correlations” which can occur without being detected by local examination of the curve fit to the calibration points. Such nonsense correlations are liable to occur in the case of polynomial response functions, which can exhibit non-monotonic behaviour with excellent local fit. Another case of nonsense correlations can occur if, by mistake, one of the calibration data uncertainties is very small. Then this calibration point is given erroneously a very high weight. Consequently, the response curve is forced through this point with little importance given to the other calibration points.
5.2.3 Examining compliance with uncertainty requirements
For the specified analytical range, an upper bound is determined for the uncertainty of the prospective results based on the analysis function. This upper bound is compared with the acceptable uncertainty.